This page hopes to define the various numbers that are seen often seen in the formulas and prescriptions for surface shapes. I'm doing this because it has been a confusing thing for me to understand as the three different values don't say exactly the same things for a particular surface shape when it comes to shapes other than a sphere or a parabola. I'm going to try to make sense of all of it in this page.
You may wish to survey this page also for some additional info on the Constants.
And then there is this page which is in mixed German and English.
In Smith's "Modern Optical Engineering", p. 392, he says a conic section through the origin satisfies the equation
And now with e = eccentricity case where:
The Aparabolic Deformation Constant is used in the BEAM 3 ray tracing program which is the only place that I've seen it used. Why, I don't know as a little bit of programming and it could have been using the (I assume as I don't have that product at hand and somebody with the program can correct me if I'm wrong and it is the eccentricity instead) Conic Constant instead.
eccentricity is defined as you take half of the distance between the foci, which equals the center-to-focus separation. So if the distance between the two foci is 6mm and the distance from one focus to the sharp end near it is 6mm then the eccentricity e is 0.5 and thus by the above formula, the Conic Constant K is -0.25. The relation between the center separation (c) on one, and horizontal (a) and vertical semiaxis (b) is given by the formula:
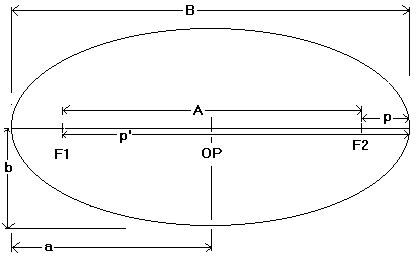
There are several ways to actually derive the e of an elipse. This drawing shows the various measurements of an elipse:

The formulas that can be used are (note the case of the letters used):
The third formula is a reformation of the second formula, deriving the values of A and B as the distance from p to the near dege of the surface is the near focus of the elipse while the p' is the distance from the far focus to the elipse. In other words, doing a concave secondary mirror for a Gregorian p is the ROC of the mirror while the p' is where the focal plane of the telescope is. A lille bit of working of these formulas will bring out all of the dimensions of the elipse.
When you look at the formulas, you'll also note that the parabola is indeed a value of 1 for e as the following indicates: